to the nearest whole ph unit, what is the ph of a 1.0 × 10–11 m hbr solution?

12.6 The pH Scale

Learning Objectives

1. Define pH.
2. Determine the pH of acidic and basic solutions.

As nosotros have seen, [H⁺] and [OH⁻] values tin can be markedly unlike from 1 aqueous solution to another. So chemists divers a new scale that succinctly indicates the concentrations of either of these two ions.

pHThe negative logarithm of the hydrogen ion concentration. is a logarithmic function of [H⁺]:

pH = −log[H⁺]

pH is usually (simply not always) between 0 and 14. Knowing the dependence of pH on [H⁺], we can summarize as follows:

• If pH < vii, then the solution is acidic.
• If pH = vii, then the solution is neutral.
• If pH > seven, then the solution is basic.

This is known equally the pH calibrationThe range of values from 0 to 14 that describes the acidity or basicity of a solution. . You lot can use pH to make a quick determination whether a given aqueous solution is acidic, bones, or neutral.

Example 12

Label each solution as acidic, basic, or neutral based just on the stated pH.

1. milk of magnesia, pH = 10.5
2. pure water, pH = 7
3. wine, pH = 3.0

Solution

1. With a pH greater than 7, milk of magnesia is basic. (Milk of magnesia is largely Mg(OH)_ii.)
2. Pure water, with a pH of vii, is neutral.
3. With a pH of less than 7, wine is acidic.

Test Yourself

Place each substance as acidic, basic, or neutral based only on the stated pH.

1. human blood, pH = 7.iv
2. household ammonia, pH = 11.0
3. cherries, pH = 3.6

Answers

1. bones
2. bones
3. acidic

Table 12.three "Typical pH Values of Diverse Substances*" gives the typical pH values of some common substances. Note that several nutrient items are on the listing, and most of them are acidic.

Table 12.iii Typical pH Values of Various Substances*

Substance	pH
stomach acid	1.7
lemon juice	2.ii
vinegar	2.9
soda	3.0
vino	3.5
coffee, blackness	5.0
milk	6.nine
pure water	seven.0
blood	7.4
seawater	8.5
milk of magnesia	10.v
ammonia solution	12.v
1.0 M NaOH	14.0
*Bodily values may vary depending on weather condition.

pH is a logarithmic calibration. A solution that has a pH of 1.0 has 10 times the [H⁺] as a solution with a pH of 2.0, which in turn has x times the [H⁺] equally a solution with a pH of 3.0 and and so forth.

Using the definition of pH, it is also possible to calculate [H⁺] (and [OH⁻]) from pH and vice versa. The general formula for determining [H⁺] from pH is every bit follows:

[H⁺] = ten^−pH

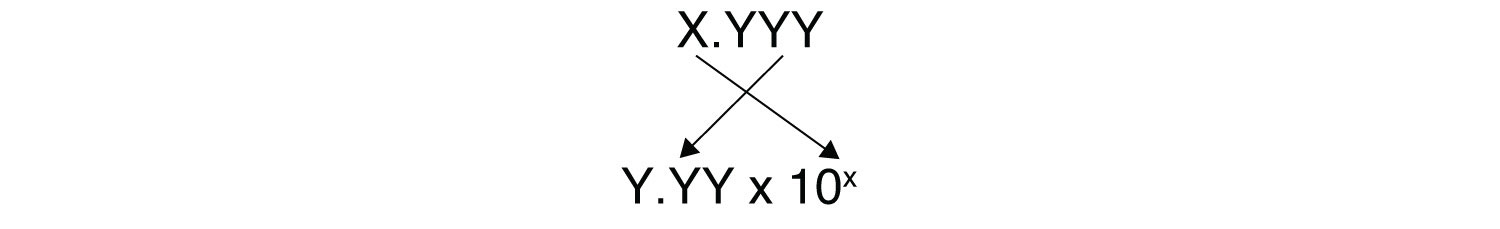
You need to determine how to evaluate the above expression on your calculator. Ask your instructor if y'all have whatsoever questions. The other issue that concerns the states here is significant figures. Considering the number(s) before the decimal betoken in a logarithm relate to the ability on ten, the number of digits after the decimal point is what determines the number of pregnant figures in the final answer:

Case 13

What are [H⁺] and [OH⁻] for an aqueous solution whose pH is 4.88?

Solution

We need to evaluate the expression

[H⁺] = 10^−iv.88

Depending on the estimator you use, the method for solving this problem volition vary. In some cases, the "−4.88" is entered and a "x¹⁰" key is pressed; for other calculators, the sequence of keystrokes is reversed. In any case, the right numerical reply is as follows:

[H⁺] = ane.iii × 10⁻⁵ M

Because 4.88 has two digits later the decimal bespeak, [H⁺] is limited to ii significant figures. From this, [OH⁻] can be determined:

$${\text{[OH}}^{-}]=\frac{\mathrm{i}\times {\mathrm{ten}}^{-14}}{1.3\times {\mathrm{ten}}^{-5}}=7.7\times {\mathrm{x}}^{-10}\text{One thousand}$$

Test Yourself

What are [H⁺] and [OH⁻] for an aqueous solution whose pH is 10.36?

Answer

[H⁺] = 4.4 × 10⁻¹¹ One thousand; [OH⁻] = 2.3 × ten^−four K

In that location is an easier manner to relate [H⁺] and [OH⁻]. We can also ascertain pOHThe negative logarithm of the hydroxide ion concentration. similar to pH:

pOH = −log[OH⁻]

(In fact, p"anything" is defined as the negative logarithm of that annihilation.) This also implies that

[OH⁻] = 10^−pOH

A unproblematic and useful relationship is that for any aqueous solution,

pH + pOH = 14

This relationship makes it elementary to determine pH from pOH or pOH from pH and then calculate the resulting ion concentration.

Example 14

The pH of a solution is 8.22. What are pOH, [H⁺], and [OH⁻]?

Solution

Because the sum of pH and pOH equals fourteen, we have

8.22 + pOH = xiv

Subtracting eight.22 from 14, we get

pOH = 5.78

Now we evaluate the following 2 expressions:

[H⁺] = 10^−8.22 [OH⁻] = 10^−five.78

So

[H⁺] = 6.0 × 10⁻⁹ G [OH⁻] = 1.7 × x^−vi Thou

Test Yourself

The pOH of a solution is 12.04. What are pH, [H⁺], and [OH⁻]?

Answer

pH = 1.96; [H⁺] = 1.1 × 10⁻² M; [OH⁻] = 9.i × 10⁻¹³ Thousand

Key Takeaways

• pH is a logarithmic office of [H⁺].
• [H⁺] tin be calculated straight from pH.
• pOH is related to pH and tin can be hands calculated from pH.

Exercises

1. Define pH. How is it related to pOH?

2. Define pOH. How is it related to pH?

3. What is the pH range for an acidic solution?

4. What is the pH range for a basic solution?

5. What is [H⁺] for a neutral solution?

6. What is [OH⁻] for a neutral solution? Compare your reply to Exercise six. Does this make sense?

7. Which substances in Tabular array 12.three "Typical pH Values of Diverse Substances*" are acidic?

8. Which substances in Tabular array 12.three "Typical pH Values of Diverse Substances*" are basic?

9. What is the pH of a solution when [H⁺] is 3.44 × x^−four One thousand?

10. What is the pH of a solution when [H⁺] is 9.04 × 10^−thirteen G?

11. What is the pH of a solution when [OH⁻] is six.22 × 10⁻⁷ M?

12. What is the pH of a solution when [OH⁻] is 0.0222 Thousand?

13. What is the pOH of a solution when [H⁺] is three.44 × 10⁻⁴ G?

14. What is the pOH of a solution when [H⁺] is nine.04 × ten^−thirteen M?

15. What is the pOH of a solution when [OH⁻] is 6.22 × 10^−seven One thousand?

16. What is the pOH of a solution when [OH⁻] is 0.0222 M?

17. If a solution has a pH of 0.77, what is its pOH, [H⁺], and [OH⁻]?

18. If a solution has a pOH of 13.09, what is its pH, [H⁺], and [OH⁻]?

Answers

1. pH is the negative logarithm of [H⁺] and is equal to fourteen − pOH.

3. pH < 7

5. one.0 × ten⁻⁷ One thousand

7. Every entry above pure water is acidic.

9. 3.46

11. 7.79

13. 10.54

15. 6.21

17. pOH = thirteen.23; [H⁺] = 1.70 × 10⁻¹ M; [OH⁻] = 5.89 × x⁻¹⁴ M

mcguiregoomil.blogspot.com

Source: https://saylordotorg.github.io/text_introductory-chemistry/s16-06-the-ph-scale.html

Share this post